String theory solution is tailor-made
Researchers cut a vexing problem into manageable shapes, and come up with a solution that could apply to all string theories.
Take a self-guided tour from quantum to cosmos!
Researchers cut a vexing problem into manageable shapes, and come up with a solution that could apply to all string theories.

A few years ago, a Brazilian-Canadian-French collaboration discovered that a particular problem in string theory could be solved if you approached it as a tailoring issue: specifically, how to cut up a pair of pants.
While it sounds like the set-up for a punchline, that sudden insight, published in 2015, has proved to be no joke. By cutting a challenging physics problem down to size, the trio of researchers found a way to simplify and solve it, and created a powerful solution for string interactions that are much too complicated to study in their full state.
Now, the tailors are at it again. In a recent paper published in the Journal of High Energy Physics, physicists from Perimeter Institute and Brazil’s Instituto de Física Teórica and the South American Institute for Fundamental Research (IFT-SAIFR) turned a string-theory problem into a pants-cutting problem, and applied it to a more complex system.
Their results address a long-standing problem in the string theory subfield of integrability, and could prove meaningful for the entire strings community.
Let’s set the scene a little. String theory posits that subatomic particles are actually strings vibrating in space. The world that we see and feel arises from how these strings move from one point to another (called propagation) and how they merge or separate (called interaction).
Integrability is the mathematical tool used to understand string propagation and interactions as they occur in a hypothetical, curved space called Anti-de Sitter (AdS) space. Why do theories in a non-realistic universe count? Because they can serve as analogies to other, more complex theories, and vice versa.
Thanks to something called the AdS/CFT duality, string theories in AdS space can be considered equivalent to other theories in a lower dimension. This means theorists are able to translate complex theories up or down to a more manageable dimension – as long as they can also translate and keep track of each theory’s unique attributes up and down the chain.

It was another analogy that finally unlocked the puzzle of string propagation. A landmark 2009 paper showed that the string propagation problem was almost equivalent to a condensed matter problem. This allowed string theorists to import and adapt condensed matter techniques.
However, there were no such analogies for string interactions. These remained a thorny challenge as researchers worked to develop new techniques with which to study them.
A powerful new idea was put forward in 2015 by Perimeter faculty member Pedro Vieira (who was a co-author on the 2009 propagation paper), Perimeter postdoc Shota Komatsu, and Benjamin Basso, then at the École Normale Supérieure – all working together in Brazil.
In what they called the “pants” approach, explained more fully below, they studied the simplest interaction of a string: when one string splits into two. Cutting the problem in half proved not only mathematically powerful, but visually helpful.
A year later, Komatsu and IFT–SAIFR postdoctoral researcher Thiago Fleury wanted to tackle a more difficult interaction: when two strings merge then split again. It turns out, they ended up finding not just a powerful way to study integrability in string theories[1] and gauge theories[2]; they might have found a new way to study all string theories.
The paper has already gained the attention of the integrability community, and will be a featured paper at the Integrability in Gauge and String Theories conference in Paris this July. It is also expected to make waves when the string theorists gather for their largest annual conference, Strings 2017, this June in Tel Aviv.
Vieira, who now holds the Clay Riddell Paul Dirac Chair at Perimeter Institute, plans to highlight the work when he provides the annual overview of research in integrability. “I think it’s really a breakthrough in understanding string interactions,” he said. “It’s definitely the most important result of the year in this field, by far.”
The strings-as-pants analogy sounds odd at first, but it makes sense when you see it. In diagram A, you see what looks like an empty pair of pants standing in front of you. At the “waist” is a closed string loop. As that string moves through time, it makes the shape of a cylinder. When the interaction occurs, and the string splits in two, it creates two new cylinders that end at the “left cuff” and the “right cuff” of the pants. This is called a three point function, because there are three “ends”.
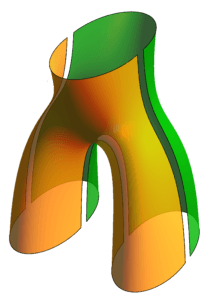
Now, take some scissors and slice down each of the outside seams, then up the inseams, to leave a “front half” and a “back half.” Each half is a hexagon: waist, left-leg seam, cuff, inseam, cuff, right-leg seam.
This theoretical tailoring means those closed strings are now sliced open, and that means they can be studied using integrability. After the strings are studied, the hexagons are then “glued” back together, with some mathematics added in to account for the introduction of “seams” where none previously existed.
The original tailoring reduced an incredibly complex computation into smaller, simpler building blocks, and provided a process through which string interactions could be understood.
This new paper, “Hexagonalization of Correlation Functions,” takes a similar approach to a different problem: how two strings interact and then split into two new strings. This is called a four-point function, because there are four “ends” of the strings. This is a much more complex situation, which means its solution can yield much richer information.
That complexity is what drew Komatsu to the problem. “Trying to understand the four-point function was a challenge. It was clear that it’s a very interesting object,” Komatsu said.
Komatsu and Fleury started by looking at results generated by studies of four-point functions in very weak regimes (where the attraction between particles is very weak, so they fly around and interact very little), and in very strong regimes (in which strong couplings create multiple interactions and make the system difficult to study).
Due to the differing math techniques used to probe each regime, the results were vastly different. But there were commonalities. At the weak end of the spectrum, the math was complicated by many contributions, but those contributions seemed to pool into four different areas. At the strong end, the strings were complicated to describe, but the math seemed to indicate that each string had four special regions.
The math was giving strong hints that the solution lay in somehow dividing the problem into four parts, but how? A number of researchers at Perimeter and SAIFR took up the challenge. Some took the pants idea, joined two “pairs” at the waist, and cut them along the seams.
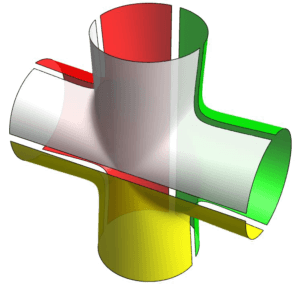
Komatsu and Fleury did something different: they cut one “side” of the interaction vertically, and the other horizontally. (See diagram B). This created four hexagons, but in a different way. It also created four split strings that could be calculated and glued back together. “The idea itself, we had it almost from the beginning,” said Komatsu, who came to Perimeter in 2014.
Using integrability, they began computing the interaction contribution from each hexagonal patch. This was a formidable effort: when the “pants” are formed, excitations called magnons are divided between the two. The researchers had to sum over all possible divisions, and to move a magnon from one hexagon to another, they had to introduce propagation phases.
Needless to say, the mathematical techniques to do these operators needed to be custom tailored. After a year collaborating from their respective home bases in Waterloo and São Paulo, and getting together during visits facilitated by the Perimeter-SAIFR partnership, Komatsu’s and Fleury’s idea was vindicated: their results using this new method agreed perfectly with previous results of the four-point function at weak couplings and at strong couplings.
Vieira says it was a powerful insight. “It’s a different way of cutting this object. You cut it into the same kind of hexagon, but the way you cut is different. It is more beautiful,” he said.

For Komatsu and Fleury, this is just the beginning. The mathematics of hexagonalization are complicated, and as the coupling strength increases, the math becomes harder to control. Both researchers are working to iron out as many issues as they can before presenting the paper at the subfield’s big conference in July.
“There are many things to understand, and to do. I’m going to work exclusively on this [until the conference],” Fleury said.
This paper provides a basic framework they look forward to expanding on, Komatsu said. “So far, we only checked that the hexagonalization can reproduce the results computed by other means,” he said. “We should in principle be able to compute many more results than are computable by other methods. To make it really work, or in order to get some real predictions, there are still some details that we need to work out.”
Because weak and strong regimes are so different, physicists use different techniques to study them. If theorists could find a single method that worked in the strong and weak regimes, they might also finally have a way to probe the regions in between, which, today, are basically impenetrable.
Vieira suspects that hexagonalization might just provide such an avenue. “I think it’s really a breakthrough in understanding string interactions,” he said.
“Because it applies to any N-point function in a very elegant way, it’s very likely that it extends beyond the gauge/gravity dualities we are studying. Shota’s and Thiago’s idea can, I suspect, be applied to all string theories.”
[1] String theory attempts to merge quantum mechanics with general relativity. String theories replace the particles in particle physics with one-dimensional strings.
[2] Gauge theory is a class of quantum field theory. Gauge theories constrain the laws of physics in a way that leaves the basic physics of the quantum field unchanged, making it possible to reveal underlying structures.
On July 27, Juan Maldacena, a luminary in the worlds of string theory and quantum gravity, will share his insights on black holes, wormholes, and quantum entanglement.
A thriving new body of research has revived an old tool – the bootstrap – and is using it as a new lens on some of the deepest and toughest problems in field theory.
Fifty years ago, surf culture reigned supreme, the summer of love was on the horizon, and a revolution started brewing – in physics. Lauren Greenspan tells the story of “the bootstrap”.